Rif Eruvin 15b {Eruvin 52b continues ... 55a}

HIDE/SHOW IMAGE
15b

{Eruvin 52b continues}
It was taught {tana}: Because of those who err in their measures.

And the halacha is like the first Tanna {that even one cubit, he may not reenter}.

Rabbi Chanina said: If his one leg was within the techum and his other leg was outside the techum, he may enter, for it is written {Yeshaya 58:13}:

יג אִם-תָּשִׁיב מִשַּׁבָּת רַגְלֶךָ, עֲשׂוֹת חֲפָצֶךָ בְּיוֹם קָדְשִׁי; וְקָרָאתָ לַשַּׁבָּת עֹנֶג, לִקְדוֹשׁ יְהוָה מְכֻבָּד, וְכִבַּדְתּוֹ מֵעֲשׂוֹת דְּרָכֶיךָ, מִמְּצוֹא חֶפְצְךָ וְדַבֵּר דָּבָר.

13 If thou turn away thy foot because of the sabbath, from pursuing thy business on My holy day; and call the sabbath a delight, and the holy of the LORD honourable; and shalt honour it, not doing thy wonted ways, nor pursuing thy business, nor speaking thereof;

which we read "your feet." {That is, it is written deficiently, in that the segol of רַגְלֶךָ would typically be accompanied by a yud, signifying the plural, and without the yud, we would expect a sheva, and it meaning foot.}

END PEREK FOUR
We have left off mi shehotziuhu.

PEREK FIVE - keitzad meabrin

Mishna:
How does one extend the bounds of the towns?
A house recedes, a house protrudes, a turret recedes, a turret protrudes; if there were there ruins ten handbreadths high,
{Eruvin 53a}
and bridges and tomb- structures which have in them dwelling quarters the measurements are taken against them, and it is made in the shape of a square board, so as to benefit from the corners.

{Eruvin 55a}
Gemara:

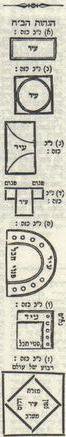
The Sages learnt {in a brayta}: How does one extend the bounds of the towns?
Long {=rectangular; see (א)}, as it is.
Circular, we make it corners {and measure techum from the corners; see (ב)}.
Squared {but on an angle from the compass directions, like a diamond, <>, such that one might think to add corners and square it in the compass directions}, we do not make it corners.
If it was wide on one side and narrow on the other side {see (ג)}, we view it as if both sides were equal {and thus add corners; Alternatively, we view the curvature as if it were straight, thus transforming it into a parallelogram.}.
If one house jutted out from it {the city} in the form of a turret, or two houses like two turrets {one one each side - see diagram ד}, we view it as if there were a line extending from each protrusion {thus squaring it} and we measure from there and outwards 2000 cubits {rather than from the city wall}.
If it {the city} was made in the form of a bow {see diagram ה - note that the line on the left side is not physically present. That is, it looks like a C} or like a gamma {A capital gamma, Γ. See diagram ו} we view it as if it {the empty space in the C or the Γ} were filled with houses and courtyards.

Mar {=the brayta} had said: Long {=rectangular, see diagram א}, as it is.
This is obvious! No, I need this. I would have thought that if it is long and narrow, I would imagine its width extended as much as its length {thus making it a square}. Therefore it informs us that it is not so.

"If it is squared {= <>}, we do not make it corners":
But this is obvious! No, I need this, for the case in which it is square but not square in the compass directions - that is, that the north flat face is parallel with the northern compass direction, and its southern flat face is parallel to the southern compass direction {but rather, it looks like diagram ז}. I would have thought to square it to the square formed by the compass directions {thus adding corners, as in diagram ז}. Therefore it informs us that it is not so.